We study the large-volume asymptotics of the sum of power-weighted edge lengths 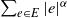 in Poisson-based spatial random networks. In the regime
in Poisson-based spatial random networks. In the regime  , we provide a set of sufficient conditions under which the upper-large-deviation asymptotics are characterized by a condensation phenomenon, meaning that the excess is caused by a negligible portion of Poisson points. Moreover, the rate function can be expressed through a concrete optimization problem. This framework encompasses in particular directed, bidirected, and undirected variants of the k-nearest-neighbor graph, as well as suitable
, we provide a set of sufficient conditions under which the upper-large-deviation asymptotics are characterized by a condensation phenomenon, meaning that the excess is caused by a negligible portion of Poisson points. Moreover, the rate function can be expressed through a concrete optimization problem. This framework encompasses in particular directed, bidirected, and undirected variants of the k-nearest-neighbor graph, as well as suitable 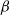 -skeletons.
-skeletons.
Mathematics
